Open Science Repository Language and Linguistics

doi: 10.7392/openaccess.70081958

Syllables and Syllable Structure in Arabic in the Light of the Optimality Theory

Ali Saleh Ibraheem Jarrah

Department of Languages and Translation, College of Arts and Humanities, Taibah University, Madina, Saudi Arabia

Abstract

The objective of this article is to explore the phonological property of syllable structure in Arabic, mainly, the Madina Hijazi Arabic (MHA), spoken in Madina Munawwarah, Saudi Arabia. The framework used in this article is the Optimality Theory (hence OT), first proposed by Prince and Smolensky (1993) and elaborated later by McCarthy and Prince  (1993a, b, 1995, and 1999). In this paper I demonstrate that a small set of constraints independently motivated for the analysis of syllable structure within Optimality Theory is sufficient to account for the gross characteristics of MHA syllable structure. This sufficiency is an important factorial typological consequence of the ranking of violable universal constraints, the cornerstone assumption of OT, a fact that serves as the primary motivating force behind this line of investigation. The constraints involved in the analysis and the logic of their interactions with one another turn out to reveal some important properties of constraint (in)activity through ranking in OT, a topic which I devote particular attention. It is argued that prosodic aspects such as syllable structure and many phonological and morphological processes are better understood as cases involving interaction between two types of conflicting universal constraints: Markedness constraints and Faithfulness constraints.

Keywords: syllables and syllable structure, phonology, optimality theory.

1. Introduction

The internal structure of the syllable has been described by many linguists in different views. Some descriptions were based on rules, templates and principles, some others were based on constraints. 

In this paper I argue that Optimality Theory (OT) Prince and Smolensky (1993), which is based on constraints, provides an explanatory model for syllable structure in Madina Hijazi Arabic (hereafter, this Arabic variety will be referred to as MHA).

Previous works on phonology assume that the task of phonological theory is to define the underlying form and surface form on a linguistic object. The matching between underlying and surface forms is achieved via phonological rules. Optimality Theory abandons the idea that the underlying (input) and surface (output) matching is accomplished via rules. Optimality Theory assigns a ranking to all of the candidate realizations of a word, calling the scale a measure of harmony. All of the candidates which show the maximal amount of harmony are accepted by the constraint system, and others are rejected. A derivation in Optimality Theory consists of an original candidate set produced by a function called GEN (Generator), and the subsequent application of constraints to reduce the candidate set, eliminate all non-optimal candidates and preserving those with greatest harmony (Prince & Smolensky 1993, McCarthy 1993, McCarthy & Prince 1993a, 1995).

2. Syllable structure before OT

The internal structure on the syllable has been described by many linguists in different views. McCawly (1968), Venneman (1988), Hooper (1976), Kahn (1976) and Clements and Keyser (1983) claim that segments on the skeletal tier are directly linked to the syllable node. Clements and Keyser, however, claim that the nucleus plays an important role in phonological representation. They propose that the phonological representation consists of the syllable tier, the CV tier, and the segmental tier. The first three tiers represent the organization of speech units at higher levels as shown in (1) below:

1.

The segmental tier, on the other hand, consists of bundles of distinctive features matrices which represent consonants and vowels.

Lowenstam (1981:593) claims that 'a syllable is maximal substring such that: 

2.

• a. no segment is lower on the hierarchy than both its immediate neighboring segments.
• b. no two segments of equal ranking on the hierarchy are adjacent. The onset is maximal within the limits of (a)'.

In this definition of syllables, no reference is made to language-specific rules. Onset is defined in the environment of well-formedness condition of syllabification.

In a second approach, the syllable is considered to have same internal constituent structure. Pike and Pike (1947) argue for the occurrence of the nucleus because phonological rules refer to it. Pike (1967) argues for the onset, peak and coda as the major constituents of the syllable. Newman (1972) recognizes the occurrence of the rime to distinguish between heavy and light syllables. McCarthy (1979a, b), Kiparsky (1979), Halle and Vergnaud (1980) introduced a hierarchical branching theory in the framework of multi-tiered phonological theory. Syllable structure in this approach is represented as follows:

3.

The syllable (σ) in the above representation consists of two constituents, namely, the onset and the rime; onset comes from the beginning and rime follows it. The rime here branches; it contains a vowel which is followed by a consonant. The rime is the head constituent, i.e., the obligatory constituent of the syllable; the onset is the sister constituent which comes from the same node (i.e., σ). 

Steriade (1982) claims that syllabic structures are constructed by rules which are ordered among the rules of phonology. She presents two types of syllabification rules, namely, universal and language specific. A universal rule parses the segmental string to form CV syllables. The language-specific rules form complex onsets and rimes (this includes branching onsets and codas). Their relative order of application, their unbounded or binary manner and the presence of segmental well-formedness conditions on their application are also language-specific.

Levin (1983, 1985) considers the nucleus as the syllable core. The coda is defined as a complement of the nucleus. The onset is defined as specifier of the syllable. McCarthy and Prince (1990) and Hayes (1982, 1986) have suggested a different proposal which represents the mora as a weigh unit. The moraic theory requires the use of the moras as a unit involved in the determination of syllable weight, such that light syllables count as monomoraic, and heavy syllables as bimoraic as shown in (4) below:

4.

McCarthy and Prince (1986, 1990) use the moraic approach to morphological templates instead of the CV- approach.

3. Previous accounts of syllable structure in MHA

Arabic syllable structure has been subject to extensive research for more than two decades. Researchers (Al-ani 1970, Al-ani and May 1973, Broselow 1976, 1979, 1980, McCarthy 1979a, b, 1980, Selkirk 1981, and Abu Salim, 1982, Jarrah 1993, Boudlal 2001, Aoun 1979, among others) have shown that the possible syllable types of the Arabic language are as in the following:

5.

•      a. CV                galam             'pen'
•      b. CVV              saakin            'resident'
•      c. CVC              jamal              'camel'       
•      d. CVVC           sakaakeen      'knives'
•      e. CVCC           darabt             'I hit'

Jarrah (1993) claims that all syllable types above exists in MHA; according to him, CV is a light syllable, CVV and CVC are heavy syllables and CVVC and CVCC are super-heavy syllables. He claims, along with Al-ani and May (1973), McCarthy (1979a. b), that the first three types are the unmarked ones in terms of their distribution, because they occur more often than the other two types (CVVC, and CVCC). CV and CVC are more frequent types, because there are no constraints of any kind on their distribution in any position in the Arabic word. They occur freely in word-initial, medial and final positions. But the CV-type is more frequent than CVC and the rest, and then the least marked and the most natural, while the CVCC syllable is much less natural or marked. On other hand, there are some constraints on the distribution of the CVV type. This type is less frequent in final position than the other two positions, and more frequent in medial position than in initial or final positions. In (6) only 'a' is acceptable syllabification but not 'b':

6.

The unacceptability of '6b' is due to the clustering of the onset position in the second syllable.

Finally, a syllable rime may contain one consonant, as in CVC and CVVC patterns, or no consonants, as in CV and CVV patterns, but not more than two consonants, and that the CVVC patterns are confined to the final position of the word. In the following representation only 'a' is acceptable but not 'b':

7.

This conforms with the concept that syllable initial and final consonants are maximized to the extent consistent with the syllable structure of the language in question.

4. Superheavy syllables 

Superheavy syllables are termed as such to distinguish CVVC and CVCC syllables from their heavy counterparts, CVV, CVC. To account for the syllabification of superheavy syllables, Jarrah (1993)  adopts Selkirk (1981) analysis of superheavy syllables which represents the superheavy string as consisting of a heavy syllable followed  by a degenerate syllable with a missing nuclear position, i.e., the extra consonant is syllabified as an onset of a syllable with an empty nucleus, as shown in (8) below:

8.

The above analysis in '8' shows that the extra consonant is syllabified as having a degenerate syllable, for both forms of superheavy syllables in MHA.

Jarrah (1993:83) concludes the following algorithm for MHA:   

9.

• a. There must be only one pre-nuclear consonant as syllable onset linked to the syllable node.
• b. The rime must contain one element or two elements at most. If the second element happens to be a consonant, link it to the rime node.
• c. If an extra consonant at the end of the word is left unsyllabified as with superheavy syllables, link it to a degenerate syllable node.

The process of syllable building is repeated until all segments are properly syllabified.

5. Syllable structure and optimality theory

Analyses within generative phonology have been stated in terms of rewrite rules (as shown above); when those rules are applied in the appropriate sequence, they produce a surface structure form from an underlying representation. Recently there has been a shift of the work on phonological analyses from rules systems to well-formedness constraints, namely the Optimality Theory ((Prince and Smolensky 1993). In their analysis, a syllable node may have a daughter Nuc and may have as leftmost or rightmost daughters respectively the nodes Ons and Cod. The nodes Ons, Nuc and Cod, in turn, may each dominate C's and V's, or may be empty.

In this section I show how Optimality Theory offers better analysis of MHA than rule and parameter approaches do.  Syllable structure in Optimality Theory is assigned to underlying forms (inputs) by a general function GEN. This is done in a single step without rules, templates or principles: any syllabification can be produced because an independently needed set of ranked and violable well-formedness constraints correctly selects the optimal form among the others.

The basic syllable structure has been viewed as of the CV type (Jakobson, 1962; Clement and Keyser, 1983). According to this statement, Prince and Smolensky (1993:85) stated the following universal constraints:

10.

• a. ONSET 

Syllables must have an onset.

• b. NO-CODA

Syllables must not have a coda.

The constraints in '10' describe what is known as the universally unmarked characteristics of the structure involved. Let's consider the input /CVCV/, which may be syllabified as in '11':

11.

• a. CVC.V
• b. CV.CV

The first syllable in '11a' violates the NOCODA constraint, which requests that syllables ends on vowels, because it is a closed syllable, i.e., ends with a consonant, so it is suboptimal. The second syllable in "11a" violates constraint '10a', namely the ONSET constraint, because it begins with a vowel. But '11b' satisfies both constraints, so it is the optimal one.

In addition to the above constraints, Prince and Smolensky (1993) suggest the PARSE and FILL constraints on syllable structure to avoid failure to incorporate segments into syllable structure:

12.

• a. PARSE

Underlying segments must be parsed into syllable structure.

• b. FILL

Syllable positions must be filled with underlying segments.

According to Prince and Smolensky, PARSE and FILL in '12', are representative of the "faithfulness family of constraints". Their functions are to constrain the relation between structure and input.  Moreover, they require that in well-formed syllables input segments are in a one-to-one correspondence with syllable positions.

McCarthy and Prince (1995, 1999) extend the reduplicative copying relation of McCarthy and Prince (1993a) to other domains where identity relations are imposed on pairs of related representations such as input and output. Later, McCarthy and Prince (1995) reformulate the faithful constraints (Prince and Smolensky 1993) to liberate them from their connection with syllabification and phonetic interpretation. They propose that the constraint FILL and part of what the constraint PARSE does be replaced by DEP and MAX, respectively, and called their theory Correspondence Theory (CT). Correspondence Theory relates representations to one another. Rankable constraints apply to correspondent elements, demanding completeness of correspondence. Correspondent segments are often identical to one another, but identity of correspondents is also enforced by ranking, and therefore violable.  The proposed constraints are formulated as follows:

13.

• a. MAX-IO

Every segment of the input has a correspondent in the output.

• b. DEP-IO

Every segment of the output has a correspondent in the input.

MAX and DEP work by the function GEN to the input form  /CVC/ as in 14:

14.

• a. CVC
• b. CV
• c. CV.Cv

(14a) parsed a (σ), but it violates the NO-CODA constraint, (14b) satisfies the NO-CODA constraint, but it violates the MAX-IO constraint since it deletes the final 'C'. (14c) Satisfies both the NO-CODA and MAX-IO because it resorted the final 'v' (which is an epenthetic vowel), but it violates the DEP-IO constraint which demands that the segments of the output have correspondents in the input.

According to Prince and Smolensky (1993), the optimal forms are those that display minimal violation of universal constraints. And since each candidate in (12) above violates one constraint, no optimal structure will be obtained among them. What works here is what McCarthy and Prince ( 1995) call ranking of constraints, in which a candidate which violates the higher ranking constraint is suboptimal, while the one that violates the lower ranking constraint is optimal.        

Ranking of constraints, according to McCarthy and Prince, is language-specific. In a language that allows codas, like MHA, the optimal candidate is (14a); as a result, the NO-CODA constraint would be ranked low in the ranking scale. In languages where MAX-IO is ranked low, (14b) would be the optimal one. And, finally, in language where DEP-IO is ranked low, candidate (14c) is the optimal. 

In conclusion, those four constraints will be applied to MHA in the following sections, and we will show their ranking and interaction according to MHA rules. 

6. MHA syllable structure and OT

To account for syllable structure for MHA, we need to apply the four universal constraints, ONSET, NO-CODA, MAX-IO, and DEP-IO mentioned in (10) and (13) above.

As shown above that MHA syllables must have an onset, I, therefore, consider the interaction of ONSET and DEP-IO. Whenever such a situation is met we appeal to epenthesis. Consider the following:

15.

In (15) above, the epenthetic element is the glottal stop /?/, and any form violating the constraint ONSET will be eliminated since there are candidate parses that meet the constraint ONSET by epenthesizing a glottal stop, thus violating the constraint DEP-IO. The items in (15) also show that ONSET must be ranked above DEP-IO. This ranking is shown in (16) below:

16.

If I reverse the ranking of ONSET and DEP-IO, the optimal candidate will be the form *[ankatab] with onsetless syllable which is not acceptable by MHA.

I need to see if the MAX-IO mentioned above interacts with ONSET and DEP-IO by adding another candidate to the above forms as in 17.

17.

The deletion of the low vowel in (17b) satisfies the constraint ONSET but violates the MAX-IO. The relation between the two in (17) makes a wrong prediction because the optimal parse is the one where the low vowel[a] of the input is deleted. This shows that the two constraints should not be ranked with respect to each other. The tableau in (18) shows the interaction of ONSET, MAX-IO, and DEP-IO.

18.

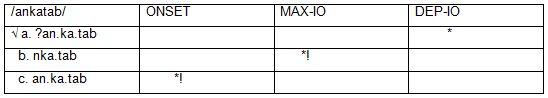
Each candidate in (18) violates one constraint, but since violation of lower ranked constraint (DEP-IO) is allowed to secure higher ranked constraints (MAX-IO and ONSET), so the optimal candidate is (18a).

The constraints above would account for glide epenthesis or the relative form in MHA as /Samawi/ 'sky like' in the tableau in (19) shows:

19.

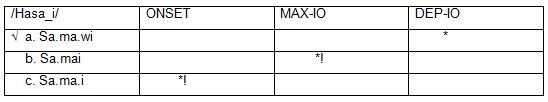
(19) Says that any violation of ONSET and MAX-IO will not be optimal.

The interaction of faithfulness constraints DEP-IO and MAX-IO with the NO-CODA constraint will be examined using the examples in (20):

20.

The items in (20) show that the NO-CODA constraint must be ranked lower in the scale than the constraint DEP-IO. Since MAX-IO dominates DEP-IO, so MAX-IO dominates NO-CODA as well, as the tableau in (21) shows:

21.

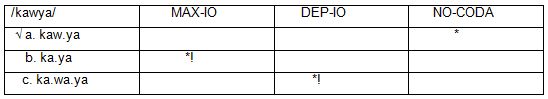
The candidate in (21b) violates the constraint MAX-IO because it deletes /w/, and the candidate in (21c) violates the constraint DEP-IO because it inserts a low vowel, and since both are higher in the rank than the NO-CODA, the candidate in (21a) is the optimal.

7. Sonority and syllabification

Sonority has been defined as the loudness of a sound relative to that of other sounds with the same length, stress and pitch (Ladefoged 1993). This means, in a syllable there is a segment constituting a sonority peak that is preceded and/ or followed by a sequence of segments with progressively decreasing value (Selkirk 1984). The sonority hierarchy is defined as follows:

22.

The sonority Sequencing Principle (SSP)

• Sonority increases towards the syllable peak and decreases towards the syllable margins.

This sonority sequencing is of limited acceptability in MHA because the number of consonants that cluster in the language is restricted to two, and they are confined to the final position of the word.   

Within the Optimality Theory, the universality of SSP and its violability is resolved in that Optimality Theory constraints are in principle violable, and patterns are derived via constraint interaction between two basic types of constraints, namely, markedness and faithfulness constraints. The SSP, which is a markedness constraint, is dominated by a faithfulness constraint that requires preservation of input clusters. There are only two levels of representation in Optimality Theory, the input and the output, and constraints are stated over output forms only. So the SSP holds at the output level.

Candidates are evaluated for harmony with respect to SSP as in the following tableau:

23.

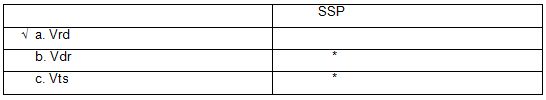
Candidate (23a) is an example of coda cluster obeying sonority generalizations. Both (23b) and (23c) are examples of clusters that violate sonority generalizations. In particular, in candidate (23b) the least sonorous segment in the cluster occurs closer to the syllable peak than the most sonorous one. This is an example of a sonority reversal. Candidate (23c) is an example of a sonority plateau, i.e., a cluster in which there is no difference in sonority between the members of the cluster, under the assumption that fricatives and stops form a single class with respect to sonority. The role that SSP plays in Optimality Theory is the core syllabification principle. It classifies clusters into two types, those that conform to the SSP and those which violate it. The candidate in (23a) is the most harmonic with respect to SSP constraint because it does not have the marks that (23b) and (23c) have. In markedness terms, this means that core clusters are the unmarked cluster type, i.e., satisfy the SSP, and both sonority plateaus and reversals are marked with respect to the SSP constraint because they violate it. 

In MHA, the sounds proceed as follows: stops, fricatives, nasals, liquids and glides. It is worth mentioning that every member of these classes may geminate, and that no clustering takes place between voiced and voiceless sounds when they share same place or manner of articulation, for example, /*td,dt/ , /*Ss, sS/, /*zs, sz/.

24.

The forms in (24) above show the possible clustering in MHA. The universals hold between core clusters and clusters that violate the SSP is captured from the interaction of Faithfulness with SSP.    

If the SSP dominates Faithfulness, only core clusters are allowed to surface because they are the only ones that satisfy the dominant SSP. This is shown in the following tableau:

25.

In tableau (25), an input containing a core cluster surfaces, despite low ranking faithfulness, because it satisfies the dominant SSP. This only allows core clusters to surface in a grammar. An input of the form /Vdr/, which is not a core cluster, will never be able to surface faithfully because of the higher ranked SSP, as shown in (26):

26.

For an input of the form /Vdr/ to surface, it is necessary that Faithfulness dominate the SSP, as shown below:

27.

In the same grammar, an input with core cluster surfaces faithfully as well:

28.

The tableau in (28) shows that if Faithfulness dominates the SSP then both types of clusters are allowed to surface. If the SSP dominates Faithfulness only core clusters are allowed to surface because they are the only harmonic clusters with respect to the markedness constraint SSP.

8. Semisyllables

Recalling the discussion of degenerate syllables and their representations in (8) above, we now turn to the discussion of degenerate syllables which are called semi or minor syllables under OT analysis. The prosodic Licensing principle formulated by Ito (1986, 1989) requires that every segment must be assigned to a higher-level constituent. Strict Layering, on the other hand, requires that every nonhighest prosodic or metrical element must be in its entirety a constituent of an element belonging to the next higher category on the prosodic hierarchy (Nespor and Vogel 1986). Higher-ranked constraints in Optimality Theory could force violation of Prosodic Licensing and strict layering. Floating elements are presumably violations of prosodic licensing, occurring when it is dominated both by markedness constraints, such as syllable, and by Faithfulness constraints. Following Selkirk (1995), I assume that strict layering corresponds to a class of subconstraints which regulate the affiliation of elements in the prosodic hierarchy. Of particular interest here will be the two constraints License-µ and license-segment, which respectively require that a mora must be affiliated with a syllable, and that a segment must be affiliated with a syllable. Ito and mester (1992, 2003) propose structures of the form in (29) for metrical constituency:

29.

Semisyllables in MHA as shown in (29) are less sonorous than syllable nuclei (in conformity with The SSP) and they are confined to the right edge of the word. The representation in (29) also shows that we need to invoke the constraint *COMPLEX-MARGIN (Prince and Smolensky 1993). This constraint is stated as follows:

30.* COMPLEX-MARGIN

• Codas and onsets must not branch.

 This constraint would be ranked above DEP-IO as the following tableau shows:

31.

I assume that MAX-IO, PARSE_SEG, and *COMPLEX are not ranked with respect to each other and that the three dominate DEP-IO. The representation in (29) also shows that there is a distinction between two types of syllables: semisyllables, and regular syllables. A semisyllable consists solely of a consonant on the periphery (right edge) of the word, whereas regular syllables are those with vowels.The grammar of MHA must have a constraint for incorporating semisyllables. This constraints is stated as follows:

32. Semi-σ

• Semisyllables are allowed in MHA.

This constraint will have to be dominated by DEP-IO, and *COMPLEX constraints to prevent epenthesis before the last consonant CVCC form, as shown in (33) below:

33.

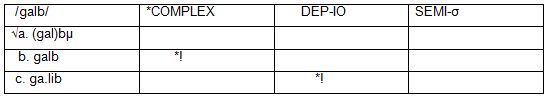
The Optimal form above is (33a) because it does not violate any constraint, while (33b) violates *COMPLEX constraint and (33c) violates the DEO-IO constraint, so  they are suboptimal

Ito and Mister (1992,2003) claim that Strict Layering does hold between moras and syllables, and formulate a principle called Mora confinement which states that µ can only be licensed by σ. In MHA, affiliation of an unsyllabified mora with the next higher category foot would violate the otherwise undominated constraint of foot size. I, therefore, will assume that it is affiliated with the prosodic word, which is not subject to any size constraint.

9. Conclusion

In this paper I have tried to account for MHA syllable structure within the Optimality Theory which is constraint based approach. It has been shown that syllabic well-formedness derived in this approach by the interaction of constraints belonging to Universal Grammar (UG) is better than rule and parameter based syllable structure building algorithm.

Two types of constraints have been distinguished, one is called dominated constraints, which are DEP-IO, NO-CODA, ALIGN-R, and the second is called undominated constraints, which are ONSET, MAX-IO, PARSE-seg, *COMPLEX and SSP. We have argued that the undominated constraints are never violated and as such they are ranked top in the ranking scale. The relative ranking of these constraints is what determines the right syllabic output.

I have also shown that in MHA sonority plays a very important role in final consonant clusters. All examples discussed above show that consonant cluster in MHA obey the SSP constraint in decreasing the sonority of the second member of the cluster to become less sonorous than the first one. 

References

1. Abu Salim, I. 1982. A Reanalysis of some aspects of Arabic phonology: A metrical approach. Ph.D. dissertation, University of Illinois at Urbana-Champagn.

2. Al-ani, S .1970. Arabic Phonology. The Hague: Mouton.

3. Al-ani,S, and & May, D 1973. The Phonological Structure of the syllable in Arabic. In Salman Al-ani , ed., Readings In Arabic Linguistics, Indiana University Linguistics Club, Bloomington, 89-101.

4. Aoun, J. 1979. Is the Syllable or the superheavy syllable a constituent? In K. Safir ed., Papers on Syllable Structure , Metrical Structure, and Harmony Processes.  MIT Working Papers in Linguistics 1. 140-148.

5. Beckman, J., Walsh L. Deckey and S. Urbanczky. 1995 (eds.). University of Massachusetts occasional papers 18:  papers in optimality Theory. Amherst, MA:GLSA.

6. Boudlal, A. 2001. Constraints Interaction in the Phonology and Morphology of Casablanca Moroccan Arabic. Ph.D. dissertation, Universite Mohammad V. Rabat, Morocco.

7. Broselow, E. 1976. The phonology of Egyptian Arabic.  Ph.D  dissertation, University of Massachusettes, Amherst.

8. Broselow, E. 1979. Cairene Arabic Syllable Structure.  Linguistics Analysis, 5, 345-382.

9. Broselow, E. 1980. Syllable structure in Two Arabic Dialects.  Studies in the Linguistic Sciences 10, 13-24.

10. Chomsky, N. & M. Halle, 1968. The Sound Pattern of English. Harper & Row , New York.

11. Clements , G.N. & S.J. Keyser 1983. CV Phonology, a Generative Theory of the Syllable. The MIT Press, Cambridge Massachusetts.

12. Dekkers, J., Van der leeuw, F, & Van De Weijer. 2000. Optimality Theory. Phonology’ Syntax, and Acquisition, Oxford Linguistics, Oxford University Press.

13. Fery, Caroline. Van de Vijver, Ruben.  2003. The Syllable in Optimality Theory. Cambridge University Press.

14. Halle, M. & J Vergnaud 1980. Three Dimensional Phonology. Journal of Linguistic research 1, 83-105.

15. Hammond, M. 1999. The Phonology of English.  A prosodic Optimality Approach, Oxford, Linguistics, Oxford University Press.

16. Hayes, B.1982. Extrametricality and English stress rules. Linguistic Inquiry 13, 227-276.

17. Hayes, B. 1986. Inalterability in CV Phonology. Language 62, 321-351.

18. Hooper, J. 1972. An Introduction to Natural Generative Phonology. New York Academic Press.

19. Ito, Junko, and Armin Mester. 1992 (2003). Weak layering and word binarity. Ms.: UC Santa Cruz. Published inTakeru Honma, Masao Okazaki, Toshiyuki Tabata and Shin-ichi Tanaka, eds. 2003. A New Century of Phonology and Phonological Theory. A Festschrift for Professor Shosuke Haraguchi on the Occasion of His Sixtieth Birthday. Tokyo: Kaitakusha. 26-65.

20. Ito, J. 1986. Syllable Theory in Prosodic Phonology. Ph.D. Dissertation, University of Massachusetts.

21. Ito, J. 1989. A Prosodic Theory of Epenthesis.  Natural Language and Linguistic Theory, 7, 217-260.

22. Jakobson, Roman. 1962. Selected Writings. The Hague.

23. Mouton Jarrah, A. 1993. The Phonology of Madina Hijazi Arabic: A Non-Linear Analysis. Ph.D. Thesis, Essex University.

24. Kager, R., H van der Hulst, & W Zonneveld (eds) 1999. The Prosody-Morphology Interface. Cambridge, Cambridge University Press.

25. Kahn, D.1976. Syllable-based Generalisations in English Phonology. Ph.D. dissertation. MIT, Massachusetts.

26. Kiparsky. P. 1979. Metrical Structure Assignment is Cyclic. Linguistic Inquiry, 10, 421-441.

27. Kiparsky, P. 2003. Syllables and Moras in Arabic. The syllable in Optimality theory ed. By Caroline Fery and Ruben van de Vijver, 47-182. Cambridge: Cambridge University Press.

28. Ladefoged, P. 1993. A Course in Phonetics.  3ed. San Diego,New York.

29. Levin, J. 1983. Reduplication and Prosodic Structure. Ms.,MIT, Cambridge.

30. Levin, J. 1985. A Metrical Theory of Syllabicity. Ph.D. dissertation, MIT, Massachusetts.

31. Lowenstamm, J. 1981. On the Maximal cluster Approach to Syllable Structure. Linguistic Inquiry 12, 575-604.

32. McCarthy, J. 1979a. Formal Problems in Semitic Phonology and Morphology. Ph.D. dissertation, MIT, Distribute by Indiana University Linguistic Club.

33. McCarthy, J. 1979b. On Stress and Syllabification. Linguistic Inquiry 10, 443-466.

34. McCarthy, J. 1980. A Note on the Accentuation of Damascene Arabic. Studies in the Linguistic sciences:10: 77-98.

35. McCarthy, J. 1997. Process-specific constraints in Optimality theory. Linguistic Inquiry 28, 231-251.

36. McCarthy, J. 2002. A thematic Guide to Optimality Theory.  Cambridge: Cambridge University Press.

37. McCarthy, J. 2003 (ed). Optimality Theory in Phonology. Cambridge, MA, Oxford, UK: Blackwell.

38. McCarthy, J. 2008. Doing Optimality Theory. Malden, MA: Wiley-Blackwell.

39. McCarthy, J & A. Prince 1986. Prosodic Morphology. MIT Press, Cambridge, Massachusetts.

40. McCarthy, J & A. Prince 1990. Foot and Word in Prosodic Morphology: The Arabic Broken Plural. Natural language and Linguistic Theory 8, 209-283.

41. McCarthy, J & A. Prince 1993a. Prosodic Morphology I: Constraint Interaction and Satisfaction. RuCCS-3, Rutgers University.

42. McCarthy , J. & A. Prince 1993b. Generalized Alignment. Yearbook of Morphology 1993, 79-153.Dordrecht: Kluwer.

43. McCarthy, J. & A. Prince 1995. Faithfulness and Reduplicative Identity. In University of Massachusetts, Occasional papers in Linguistics 18: papers in Optimality Theory, ed. Jill Beckman, Laura Walsh Dickey, and Suzanne   Urbanczyk, 249-384. Amherst, MA: GLSA publications, Rutgers Optimality Archive 60.

44. McCarthy, J & A. Prince 1999. Faithfulness and Identity in Prosodic Morphology. In The Prosody-Morphology interface, ed. Rene Kager, Harry van der Hulst and Wim Zonneveld. Cambridge. MA: MIT press, 218-309.

45. McCawly, J 1968. The Phonological Component of a Grammar of Japanese. The Hague mouton.

46. Nespor, M. & I. Vogel 1986. Prosodic Phonology. Dordrecht Foris.

47. Newman, P. 1972. Syllable Weight as a Phonological variable. Studies in African Linguistics 3, 301-323.

48. Pike, K. 1967. Language in Relation to a Unified Theory of the Structure of Human Behavior. The Hague mouton.

49. Pike, K. & E. Pike. 1947. Immediate Constituents of Mazateco Syllables. International Journal of American Linguistics.

50. Prince, A & P. Smolensky 1993. Optimality Theory: Constraint Interaction in Generative Grammar. Technical report no.RuCCS-TR-2. New Brunswick, NJ: Rutgers University Center for Cognitive Science.

51. Selkirk, E. 1981. Epenthesis and Degenerate Syllable in Cairene Arabic. In H. Borer and Y Aoun eds.,Theoretical Issues in the Grammar of Semitic Languages. MIT Working Papers inLinguistics 3, 209- 232.

52. Selkirk, E. 1984. On the Major Class Features and Syllable Theory. In M. Aronof and R. Oehrle eds., LangaugeSound Structure: Studies in Phonology presented to Morris Halle by his teacher and students. Cambridge : MIT Press.

53. Selkirk. E. 1995. The Prosodic Structure of Function Words. In University of Massachusetts Occasional papers in Linguistics 18: Papers in Optimality Theory, ed. Jill Becman , Laura Walsh Dickey, and Suzanne Urbanczky, 439-469. Amherst, MA:GLSA publications.

54. Steriade, D. 1982. Greek Prosodies and The Nature of Syllabification. Ph.D. Dissertation. MIT.

55. Vennemann, T. 1988. Preference Laws for Syllable Structure and the explanation of Sound Change. Berlin, Mouton de, Gruyter.

Cite this paper

APA

Jarrah, A. S. I. (2013). Syllables and Syllable Structure in Arabic in the Light of the Optimality Theory. Open Science Repository Language and Linguistics, Online(open-access), e70081958. doi:10.7392/openaccess.70081958

MLA

Jarrah, Ali Saleh Ibraheem. “Syllables and Syllable Structure in Arabic in the Light of the Optimality Theory.” Open Science Repository Language and Linguistics Online.open-access (2013): e70081958.

Chicago

Jarrah, Ali Saleh Ibraheem. “Syllables and Syllable Structure in Arabic in the Light of the Optimality Theory.” Open Science Repository Language and Linguistics Online, no. open-access (May 14, 2013): e70081958. http://www.open-science-repository.com/syllables-and-syllable-structure-in-arabic-in-the-light-of-the-optimality-theory.html.

Harvard

Jarrah, A.S.I., 2013. Syllables and Syllable Structure in Arabic in the Light of the Optimality Theory. Open Science Repository Language and Linguistics, Online(open-access), p.e70081958. Available at: http://www.open-science-repository.com/syllables-and-syllable-structure-in-arabic-in-the-light-of-the-optimality-theory.html.

Science

1. A. S. I. Jarrah, Syllables and Syllable Structure in Arabic in the Light of the Optimality Theory, Open Science Repository Language and Linguistics Online, e70081958 (2013).

Nature

1. Jarrah, A. S. I. Syllables and Syllable Structure in Arabic in the Light of the Optimality Theory. Open Science Repository Language and Linguistics Online, e70081958 (2013).

doi

Research registered in the DOI resolution system as: 10.7392/openaccess.70081958.

This work is licensed under a Creative Commons Attribution 3.0 Unported License.